Taylor series for \(f_\theta (r)\) near r = 0 (for bottom part of tree)

Recall that \(f_\theta (r) = y_m - y_k\) where y_k is the y-coordinate of the branch tip for the path R^k(LR)^∞ and y_m is the y-coordinate of the branch tip for the path R^m(LR)^∞, where k is the smallest integer such that kθ ≥ 180° and m is the smallest integer such that mθ ≥ 540. Note that k ≥ 2 and m ≥ 4. In fact, m ≥ k+2. For indeed, if m = k+1, then \[m\theta = (k+1)\theta = (k-1)\theta + 2\theta < 180^\circ + 2\theta < 180^\circ + 360^\circ = 540^\circ \] which is a contradiction. Also kθ < 360° since \[ k\theta ≥ 360^\circ \Rightarrow (k-1)\theta = k\theta - \theta ≥ 360^\circ - \theta > 360^\circ - 180^\circ = 180^\circ \] which is again a contradiction.

Let \(\alpha = e^{\theta i}\). The branch tip for R^k(LR)^∞ occurs at the point corresponding to the complex number \[ z_k = \left(i+ir\alpha^{-1} + ir^2\alpha^{-2} + ir^3\alpha^{-3} \ldots + ir^k\alpha^{-k} \right)+ \left(ir^{k+1}\alpha^{-k+1} + ir^{k+2}\alpha^{-k} + \ldots\right). \] Then \[ \begin{align} y_k = \text{Im}(z_k) &= 1 + r\cos(\theta) + r^2\cos(2\theta) + r^3\cos(3\theta) + \ldots + r^k\cos(k\theta) \\ \\ &\quad\quad+ r^{k+1}\cos((k-1)\theta) + r^{k+2}\cos(k\theta) + \ldots. \end{align} \] The value of y_m is obtained in the same way to get \[ \begin{align} y_m = \text{Im}(z_k) &= 1 + r\cos(\theta) + r^2\cos(2\theta) + r^3\cos(3\theta) + \ldots + r^k\cos(k\theta) \\ \\ &\quad\quad+ r^{k+1}\cos((k+1)\theta) + r^{k+2}\cos((k+2)\theta)\\ \\ &\quad\quad+ \dots + r^m\cos(m\theta) + r^{m+1}\cos((m-1)\theta) + r^{m+2}\cos(m\theta) + \ldots. \end{align} \] Subtracting the two expressions for the y-coordinates shows that \[ \begin{align} f_\theta (r) &= r^{k+1}\left(\cos((k+1)\theta) - \cos((k-1)\theta)\right) + r^{k+2}\left(\cos((k+2)\theta) - \cos(k\theta)\right) + \ldots \\ \\ &= -2\sin(\theta)\sin(k\theta)r^{k+1} + \left(\cos((k+2)\theta) - \cos(k\theta)\right)r^{k+2} + \ldots. \end{align} \]

Suppose kθ > 180°. Since 180° < kθ < 360° we have \(\sin(\theta) > 0\) and \(\sin(k\theta) < 0\), and so the coefficient of r^k+1 will be positive, and in particular, non-zero. Therefore \(f_\theta (r)\) will behave like the function \({-2\sin(\theta)\sin(k\theta)}r^{k+1}\) when r is close to 0.

If kθ = 180°, then the r^k+1 term in the formula for \(f_\theta (r)\) will cancel. Therefore the behavior of \(f_\theta (r)\) will be determined by the r^k+2 term when r is small. Hence \(f_\theta (r)\) will behave like the function \[ \left(\cos((k+2)\theta) - \cos(k\theta)\right)r^{k+2} = \left(-\cos(2\theta) + 1\right) r^{k+2} . \] Again the coefficient will be positive. It is important to note that because m ≥ k+2, no term involving a power of r with exponent greater than or equal to m+1 will affect the r^k+1 or r^k+2 terms.

So in either case, when r is close to 0, the function \(f_\theta (r)\) will behave like Arⁿ with A > 0. Thus the graph of \(f_\theta (r)\) will be increasing and concave up for small values of r.

Examples

Asking about the behavior of a function near r = 0 is really asking about the Taylor series for the function centered at r = 0. A computer algebra system like Maple or Mathematica can calculate a Taylor series up to a given order.

Example 1: θ = 135° (so k = 2 and m = 4, with kθ > 180°)

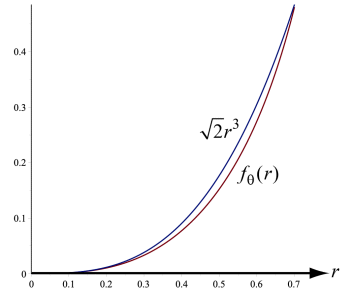\[ f_\theta (r) = \sqrt{2}r^3 - r^4 + \sqrt{2}r^5 + \ldots \]

Example 2: θ = 60° (so k = 3 and m = 9, with kθ = 180°)

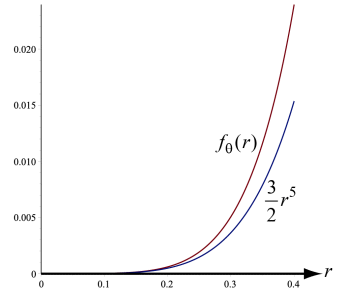\[ f_\theta (r) = \frac{3}{2}r^5 + \frac{3}{2}r^6 + \frac{3}{2}r^7 \]

In this example \(f_\theta (r)\) is actually equal to the 7th degree polynomial.