Proofs for Pedal Triangles

In the following diagram, the points A₁, B₁, and C₁ are where the altitudes from each vertex of the acute triangle ΔABC intersect the opposite sides. Each of these altitudes makes an angle of 90° with the side of the triangle.

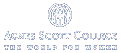
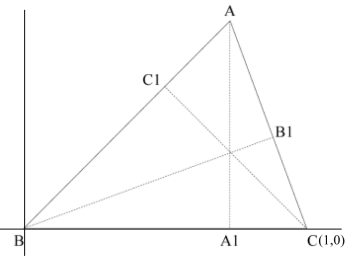
We want to show that triangles ΔAB₁C₁, ΔA₁BC₁, and ΔA₁B₁C are similar to triangle ΔABC. We will do this for triangle ΔAB₁C₁. The argument for the other two triangles are similar. It is only necessary to show that triangles ΔABC and ΔAB₁C₁ have the same angles. Since the vertex at A is common to both triangles, that angle is the same for both triangles. We now show that ∠B = ∠AB₁C₁.

First, notice that ∠AEC₁ = ∠B because ΔABA₁ and ΔAC₁E are both right triangles that share the angle ∠C₁AE.

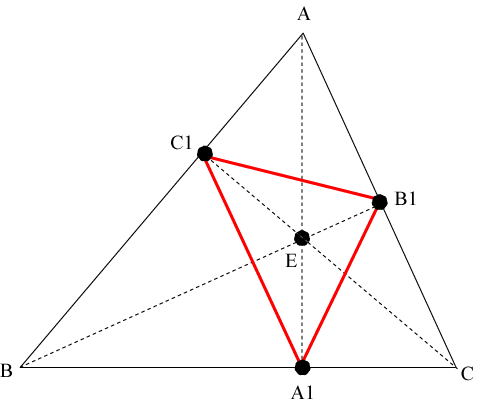
Let b = ∠AEC₁ and m = ∠AB₁C₁. We want to show that b = m. In the figure above, the three points A, C₁, and E lie on a circle. Because ΔAC₁E is a right triangle, the segment AE is a diagonal of this circle. Now the three points A, B₁, and E also lie on a circle. Since ΔAB₁E is also a right triangle, the segment AE is a diagonal of this circle. Thus both circles must be the same since they have the same diagonal. Thus all four points actually lie on the same circle as shown in the figure.
 

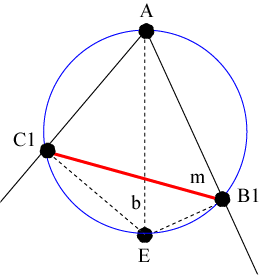
Lemma: Let points P, R, and S lie on a circle with center O. In the figure below, if the chords SR and SP form the same arc as the chords OR and OP, then the central angle n is twice the inscribed angle s.

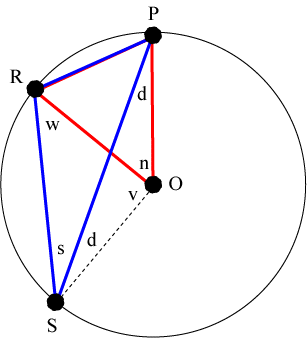
To see why this is true, first notice that triangles ΔORS and ΔOPR are isosceles triangles. Also observe that 2d+n+v = 180° and 2w+v = 180° (since s+d = w). Subtracting these two equations shows that 2(d-w) + n = 0, or 2(w-d) = n. But w-d = s, so we get 2s = n.

 
Now apply the lemma to the situation above. Since both sets of chords from points E and B₁ form the same arc of the circle AC₁, the lemma implies that both angles are half the central angle with that arc, and hence b = m.

Consider again the picture of the pedal triangle. We want to know the scaling factor relative to the original triangle for each of the similar triangles left when the pedal triangle is removed .

Since ΔABC is similar to ΔAB₁C₁, the corresponding sides are proportional, i.e.

\[\frac{{A{B_1}}}{{AB}} = \frac{{A{C_1}}}{{AC}} = \frac{{{B_1}{C_1}}}{{BC}}\]

But ΔABB₁ is a right triangle, so cos(A) = AB₁/AB. Thus the scaling factor for the top triangle is cos(A). The same argument shows that the scaling factor for the lower left triangle is cos(B) and the scaling factor the lower right triangle is cos(C).

The IFS for the Sierpinski pedal triangle involves scaling, reflections, rotations, and translations. We can take the original triangle so that vertex B is at the origin and vertex C is at the point (1,0). The triangle is then completely specified by the values of the three angles A, B, and C.

Consider the following example.

 

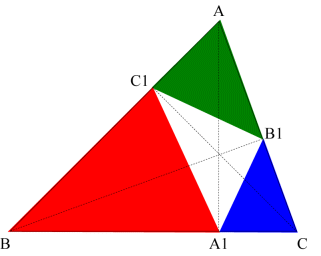
The scaling factor, as shown above, for the red triangle is cos(B). The first step is to scale the original triangle by cos(B).

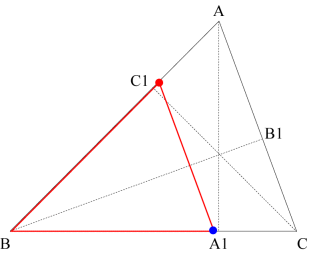
Triangle ΔABC is similar to triangle ΔA₁BC₁ as shown in the first section above. Thus the red point must go to point A₁ and the blue point must go to C₁. To do this, first do a vertical reflection across the x-axis followed by a counterclockwise rotation through the angle B. No additional translation is needed.

The scaling factor for the blue triangle is cos(C). Scale the original triangle by cos(C).

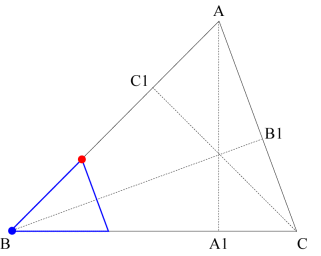
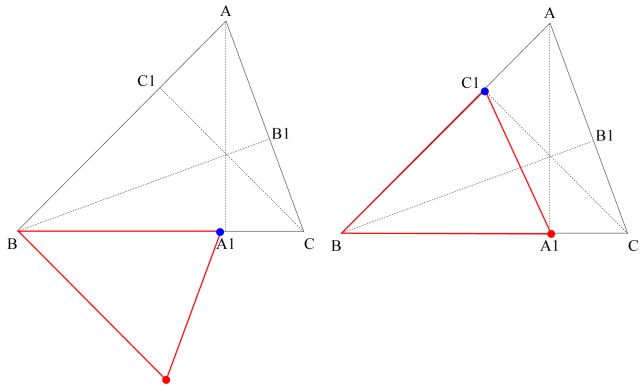
Triangle ΔABC is similar to triangle ΔA₁B₁C. Thus the red point must go to A₁ and the blue point must go to B₁. To do this, first do a vertical reflection across the x-axis followed by a clockwise rotation through the angle C.

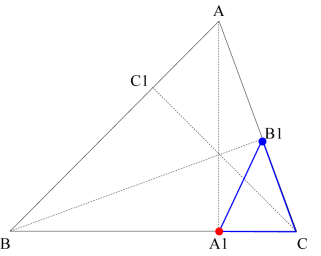
The final step is to translate the triangle so that the blue point goes to B₁. To find the coordinates (x,y) of B₁, first notice that \({B_1}C = BC \cdot \cos (C) = \cos(C) \) and therefore

\[\begin{align} x &= 1 - {B_1}C \cdot \cos (C) = 1 - {\cos ^2}(C) = {\sin ^2}(C) \\ y &= {B_1}C \cdot \sin (C) = \sin (C)\cos (C) \\ \end{align}\]

The scaling factor for the green triangle is cos(A). Scale the original triangle by cos(A).

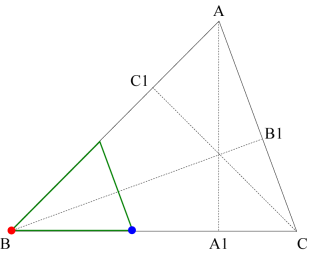
Triangle ΔABC is similar to triangle ΔAB₁C₁. Thus the red point must go to B₁ and the blue point must go to C₁. To do this, first do a horizontal reflection across the y-axis.

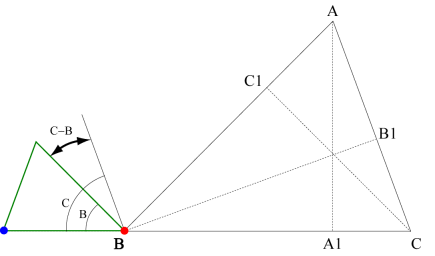
Now we need to rotate the green triangle so that the side from the top vertex to the origin becomes parallel to side AC. As the following figure shows, this involves rotating the green triangle clockwise through the angle C−B. (If C < B, then the rotation would be counterclockwise through the angle B−C.)

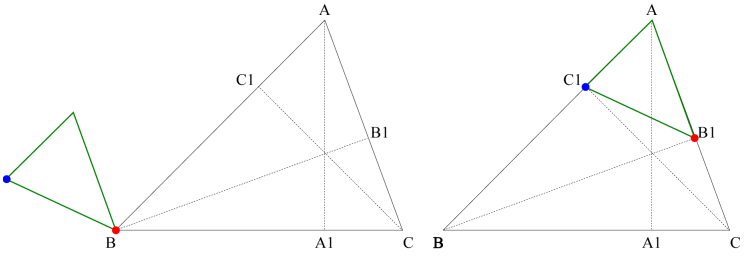
The final step is to translate the green triangle so that the red point goes to point B₁. This translation is the same as for the blue triangle.

Note: If the length of line segment BC is not equal to 1, then the translation vector should be multiplied by the length of segment BC.

The following video shows an animation that summarizes the steps in the iterated function system for generating a Sierpinski pedal triangle.

 

1. Xin-Min Zhang, Richard Hitt, Bin Wang, and Jiu Ding. "Sierpinski Pedal Triangles," Fractals, Vol. 16, No. 2 (2008), 141-150 [Available at http://www.richardhitt.com/research/hittzhang.pdf]
2. Hobson, Ernest William. A Treatise on Plane Trigonometry, 6th Edition, Cambridge University Press, 1925, p197-198.