Details about Golden 144° Symmetric Binary Trees

The five trees are placed so that their trunks start at the origin and are of length 1. The scaling factor is \(r = r_{sc} = \frac{1}{\phi}\) where \(\phi\) is the golden ratio. It is useful to note that \[ \phi^2 = \phi+1 \Rightarrow 1 = \frac{1}{\phi} + \frac{1}{\phi^2} \Rightarrow 1-\frac{1}{\phi^2} = \frac{1}{\phi} \Rightarrow 1-r^2 = r. \] and that \(\phi = 2\cos(36^\circ)\).

Let \(\alpha = e^{144^\circ i}\). This complex number represents a counterclockwise rotation of 144°. The complex number \(\alpha^{-1}\) represents to a clockwise rotate of 144°.

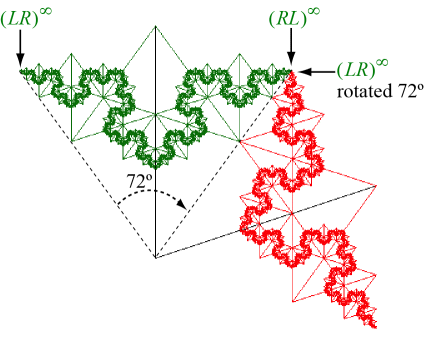
Using that \(1-r^2 = r\), we can see that the branch tip for the path (RL)^∞ in the green tree corresponds to the complex number \[ \begin{align} i + ir\alpha^{-1} + ir^2 \alpha &+ ir^3 \alpha^{-1} + ir^4 + ir^5 \alpha^{-1} + ir^6 + ir^7 \alpha^{-1} + \cdots \\ \\ &= \frac{i}{1-r^2} + \frac{ir\alpha^{-1}}{1-r^2} = \frac{i}{r} + i\alpha^{-1} \\ \\ &= i\phi + i\left(\cos(144^\circ) - i\sin(144^\circ)\right) = \sin(36^\circ) + i\left(\phi - \cos(36^\circ)\right) \\ \\ &= \sin(36^\circ) + i\cos(36^\circ) . \end{align} \] Similarly, the branch tip for the path (LR)^∞ in the green tree corresponds to the complex number \[ \begin{align} i + ir\alpha + ir^2 \alpha &+ ir^3 \alpha + ir^4 + ir^5 \alpha + ir^6 + ir^7 \alpha + \cdots \\ \\ &= \frac{i}{1-r^2} + \frac{ir\alpha}{1-r^2} = \frac{i}{r} + i\alpha \\ \\ &= i\phi + i\left(\cos(144^\circ) + i\sin(144^\circ)\right) = -\sin(36^\circ) + i\left(\phi - \cos(36^\circ)\right) \\ \\ &= -\sin(36^\circ) + i\cos(36^\circ) . \end{align} \] Rotating this last branch tip point by 72° clockwise corresponds to multiplying the complex number for the branch tip by \(\alpha^{-1}\). This means the rotated point becomes \[ \begin{align} &\left(-\sin(36^\circ) + i\cos(36^\circ)\right)\left(\cos(72^\circ) - i\sin(72^\circ)\right) \\ \\ &= \sin(72^\circ)\cos(36^\circ) - \sin(36^\circ)\cos(72^\circ) + i\left(\cos(72^\circ)\cos(36^\circ) + \sin(72^\circ)\sin(36^\circ)\right) \\ \\ &= \sin(72^\circ - 36^\circ) + i \cos(72^\circ - 36^\circ) \\ \\ &= \sin(36^\circ) + i\cos(36^\circ) \end{align} \] which corresponds to the branch tip (RL)^∞. Since the green golden tree is rotated by 72° clockwise to get the red golden tree, this shows that the green golden tree and the red golden tree just touch at this common branch point. By symmetry, the same thing happens for the other pairs of trees.